📚 Cube Numbers from 1 to 30 – Comprehensive Guide for Exam Preparation with cubes from 1 to 30
🧠 Understanding Cube Numbers
A cube number is the result of multiplying a number by itself three times. Mathematically, it’s expressed as
Cube of n = n × n × n = n³
For example, the cube of 2 is calculated as
2 × 2 × 2 = 8
Understanding cube numbers is essential for various mathematical applications, including algebra, geometry, and problem-solving in competitive exams.
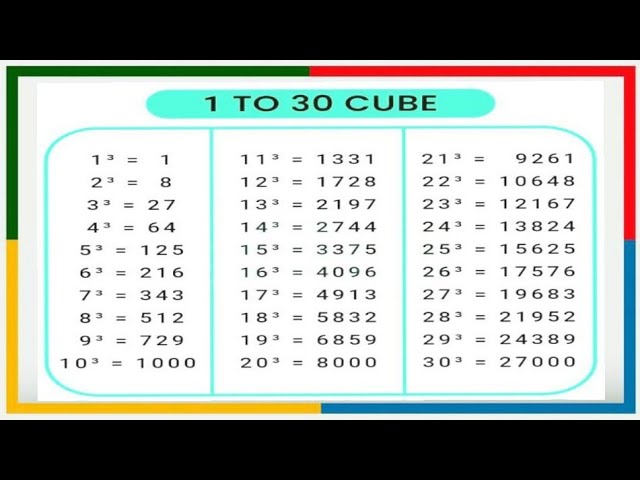
📋 Cube Numbers from 1 to 30
Here’s a complete and easy-to-read table listing the cube numbers 1 to 30:
| Number | Cube (n³) |
| 1 | 1 |
| 2 | 8 |
| 3 | 27 |
| 4 | 64 |
| 5 | 125 |
| 6 | 216 |
| 7 | 343 |
| 8 | 512 |
| 9 | 729 |
| 10 | 1000 |
| 11 | 1331 |
| 12 | 1728 |
| 13 | 2197 |
| 14 | 2744 |
| 15 | 3375 |
| 16 | 4096 |
| 17 | 4913 |
| 18 | 5832 |
| 19 | 6859 |
| 20 | 8000 |
| 21 | 9261 |
| 22 | 10648 |
| 23 | 12167 |
| 24 | 13824 |
| 25 | 15625 |
| 26 | 17576 |
| 27 | 19683 |
| 28 | 21952 |
| 29 | 24389 |
| 30 | 27000 |
This list of cubes from 1 to 30 is extremely helpful for exam-takers and school students aiming to build a strong foundation in mathematics.
🎯 Why You Should Learn Cubes 1 to 30
Memorizing cubes 1 to 30 gives you a strong advantage in:
- Quick Calculations: Speeds up your math-solving ability.
- Competitive Exams: Frequently appear in exams like SSC, Banking, UPSC, and others.
- Algebra & Geometry: Essential in solving equations involving the cube of 1 to 30 and understanding volume.
📝 How to Memorize the Cube of 1 to 30 Easily
- Use Patterns: Notice how some cubes end with the same digit as their base number.
- Flashcards: Create flashcards with numbers on one side and cubes on the other.
- Practice Tests: Take short quizzes daily.
- Group Practice: Recite 1 to 30 cubes with a study buddy.
🔍 Use these search terms to Find Learning Resources
To find the best learning tools online, you can search using these keywords:
- cubes from 1 to 30
- cubes 1 to 30
- 1 to 30 cubes
- cube of 1 to 30
- cube numbers 1 to 30
These keywords lead to high-quality worksheets, YouTube tutorials, apps, and free PDFs for effective practice.
📘 Bonus Tip: Learn Cube Roots Too
Understanding cube roots is just as important, especially in solving reverse problems in exams. Once you’re confident with cubes from 1 to 30, move on to cube roots to complete your prep.
✅ Final Thoughts
Mastering the cubes is a small step that brings a big boost to your confidence in math exams. Bookmark this page, practice regularly, and soon the cube of 1 to 30 will be second nature to you.
Keep learning, and stay ahead in your exam preparation!
X+X+X+X is equal to 4x
The equation “x+x+x+x is equal to 4x” represents a basic algebraic concept. When you add the variable “x” four times, you get 4 times the value of x, expressed as 4x. This process follows the rules of algebraic addition, where you combine like terms. For example, if x equals 2, then x+x+x+x would be 2+2+2+2, which equals 8, or 4 times 2, written as 4x. Understanding equations like “x+x+x+x is equal to 4x” is fundamental to mastering more advanced algebraic concepts.