Understanding the Dimensional Formula of Surface Tension
Surface tension is a physical property that describes the force that acts on the surface of a liquid leading to the minimization of its surface area. The dimensional formula for surface tension can be derived from its definition, which is force per unit length. This means that the units for surface tension are force divided by length, which can be represented as N/m or dyn/cm in the International System of Units (SI) or the CGS system, respectively.
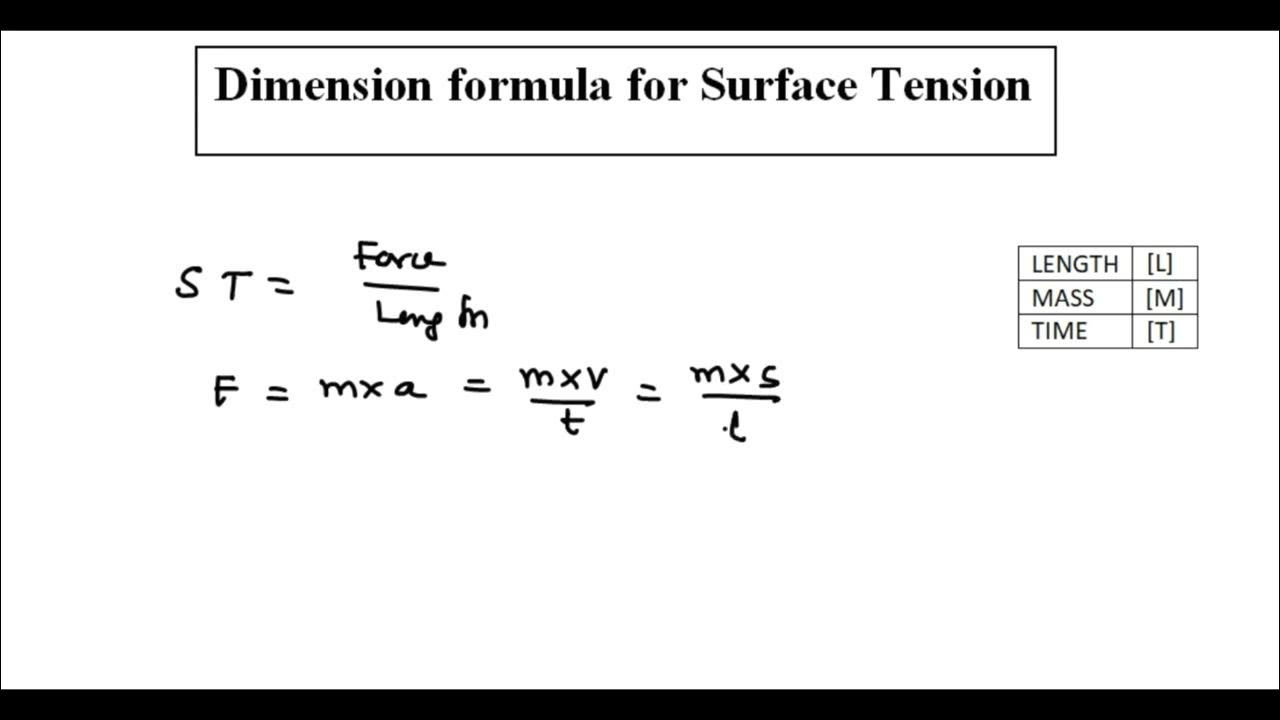
By breaking down the dimensional formula for surface tension, we can see that the dimensions for force are [M L T^-2] (Mass x Length x Time^-2) and for length are [L] (Length). Therefore, combining these dimensions gives the dimensional formula for surface tension as [M T^-2]. This means that surface tension is an inherent property of liquids that depends on the mass and time components involved in the force that acts to reduce the surface area of the liquid.
Understanding the dimensional formula of surface tension is important in physics and engineering as it helps in quantifying and predicting the behavior of liquids at their interfaces. By understanding the units and dimensions involved in surface tension, scientists and engineers can better analyze the forces at play and develop strategies to manipulate surface tension for various applications such as in the fields of fluid dynamics, microfluidics, and materials science.
Derivation and Explanation of the Dimensional Formula of Surface Tension
Surface tension is defined as the force per unit length acting perpendicular to the surface of a liquid. The dimensional formula of surface tension can be derived by equating the dimensions of force and length in the formula for surface tension (T = F/l), where T is the surface tension, F is the force acting perpendicular to the surface, and l is the length along the surface.
By applying the dimensions of force (MLT^-2) and length (L) to the formula for surface tension, we find that the dimensional formula for surface tension is [MT^-2]. This means that the surface tension of a liquid is directly proportional to the force acting perpendicular to its surface and inversely proportional to the length along the surface.
Understanding the dimensional formula of surface tension helps in the study and analysis of various phenomena such as capillary action, droplet formation, and the behavior of liquid surfaces. It is an important parameter in determining the stability and behavior of liquids in different contexts, such as in industrial processes, biological systems, and material science.
Significance of Knowing the Dimensional Formula of Surface Tension
Knowing the dimensional formula of surface tension is significant as it helps in understanding the physical properties and behavior of liquid surfaces. Surface tension is a crucial property that influences various phenomena such as capillary action, droplet formation, and the shape of liquid surfaces. By knowing the dimensional formula, one can determine how different factors like temperature, pressure, and surface area affect surface tension.
Furthermore, studying the dimensional formula of surface tension can aid in calculations and problem-solving in physics and engineering. Understanding the units and dimensions involved in surface tension can help in determining the relationships between different variables and in deriving equations for various applications. This knowledge is essential for designing experiments, analyzing data, and predicting the behavior of liquids in different scenarios.
Overall, knowing the dimensional formula of surface tension is important for gaining insights into the underlying principles governing the behavior of liquid surfaces. This understanding can lead to advancements in various fields such as materials science, fluid dynamics, and biophysics, where surface tension plays a crucial role in shaping the behavior of liquids at interfaces.
Comparison of Dimensional Formula of Tension and Surface Tension
The dimensional formula of tension is [MLT^-2], where M represents mass, L represents length, and T represents time. Tension is a force that is transmitted through a string, rope, cable, or wire when it is pulled tight by forces acting from opposite ends. It is an essential concept in mechanics and engineering, as it plays a crucial role in determining the strength and stability of structures.
On the other hand, the dimensional formula of surface tension is [MT^-2], where M represents mass and T represents time. Surface tension is a property of liquids that results from the cohesive forces between the liquid molecules at the surface. It is responsible for phenomena such as capillary action, droplet formation, and the shape of liquid surfaces. Surface tension is an important factor in understanding the behavior of fluids, especially in applications such as chemistry, biology, and engineering.
While tension and surface tension both involve forces and have similar dimensional formulas, they operate in different contexts and have distinct effects. Tension relates to the force exerted on a material when it is stretched or pulled, while surface tension involves the cohesive forces between molecules at the surface of a liquid. Both concepts are vital in understanding the physical properties of materials and play crucial roles in various scientific and engineering disciplines.
Key Concepts Related to the Dimensional Formula of Surface Tension
Surface tension is a physical property of liquids that arises from the cohesive forces between molecules at the surface of the liquid. It is measured in units of force per unit length, such as N/m or dyn/cm. The dimensional formula of surface tension is [MT-2].
The surface tension of a liquid is influenced by factors such as temperature, the presence of impurities, and the nature of the liquid molecules. Higher surface tension indicates stronger intermolecular forces, which can lead to phenomena like capillary action and the formation of droplets. Surface tension plays a crucial role in various natural processes such as the way water striders can glide across the surface of water due to the high surface tension of water molecules.
Understanding the dimensional formula of surface tension helps in quantifying and expressing this physical property in mathematical terms. By analyzing the dimensions of surface tension, we can better understand its relationships with other physical quantities and how it may change under different conditions. The dimensional formula serves as a useful tool in physics for calculating and predicting the behavior of surface tension in various systems and applications.
