Integration Formula

Integration Formula

Integration is one of the most important concepts in calculus. It can be understood as the reverse process of differentiation, and is also called inverse differentiation. In simple terms, integration is the process of finding a function when its derivative is known.

Learning the basic integration formula is essential for solving a wide range of mathematical problems in physics, engineering, and higher mathematics. This article provides:

A list of standard integral formulas
A classification of integral formulas
Integration formula for different types of functions
Practice problems to help you master these formulas

📑 Table of Contents

List of Basic Integral Formulas
Integral Formulas PDF
Classification of Integral Formulas
Integral Formulas for Different Functions
4.1 Rational Functions
4.2 Irrational Functions
4.3 Trigonometric Functions
4.4 Inverse Trigonometric Functions
4.5 Hyperbolic Functions
4.6 Exponential Functions
4.7 Logarithmic Functions
Integral Formulas for Special Functions
Practice Problem Using Integral Formulas

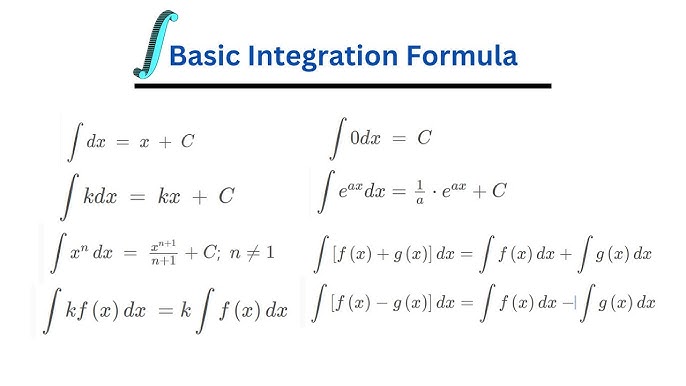
1. List of Basic Integral Formulas

These are the most commonly used basic integration formula:

∫ 1 dx = x + C
∫ a dx = ax + C
∫ xⁿ dx = (xⁿ⁺¹)/(n+1) + C ; n ≠ -1
∫ (1/x) dx = ln|x| + C
∫ eˣ dx = eˣ + C
∫ aˣ dx = (aˣ / ln a) + C ; a > 0, a ≠ 1
∫ sin x dx = -cos x + C
∫ cos x dx = sin x + C
∫ sec²x dx = tan x + C
∫ csc²x dx = -cot x + C
∫ sec x·tan x dx = sec x + C
∫ csc x·cot x dx = -csc x + C

2. Integral Formulas PDF

You can compile all the formulas listed in this article into a single PDF for quick reference and revision.
(This section can link to your downloadable resource file if you host one.)

3. Classification of Integral Formulas

Integration formulas can be grouped based on the type of functions involved:

Rational functions
Irrational functions
Trigonometric functions
Inverse trigonometric functions
Hyperbolic functions
Inverse hyperbolic functions
Exponential functions
Logarithmic functions
Gaussian functions
Special functions

4. Integral Formulas for Different Functions

4.1 Rational Functions

∫ 1 dx = x + C
∫ a dx = ax + C
∫ (1/x) dx = ln|x| + C

4.2 Irrational Functions

Examples: ∫ √x dx, ∫ 1/√x dx, etc.

∫ x^(1/2) dx = (2/3) x^(3/2) + C
∫ x^(-1/2) dx = 2x^(1/2) + C

4.3 Trigonometric Functions

∫ sin x dx = -cos x + C
∫ cos x dx = sin x + C
∫ sec²x dx = tan x + C
∫ csc²x dx = -cot x + C
∫ sec x·tan x dx = sec x + C
∫ csc x·cot x dx = -csc x + C

4.4 Inverse Trigonometric Functions

∫ (1/√(1 – x²)) dx = sin⁻¹x + C
∫ (-1/√(1 – x²)) dx = cos⁻¹x + C
∫ (1/(1 + x²)) dx = tan⁻¹x + C
∫ (-1/(1 + x²)) dx = cot⁻¹x + C
∫ (1/(|x|√(x² – 1))) dx = sec⁻¹x + C
∫ (-1/(|x|√(x² – 1))) dx = cosec⁻¹x + C

4.5 Hyperbolic Functions

∫ sinh x dx = cosh x + C
∫ cosh x dx = sinh x + C
∫ sech²x dx = tanh x + C
∫ csch²x dx = -coth x + C

4.6 Exponential Functions

∫ eˣ dx = eˣ + C
∫ aˣ dx = (aˣ / ln a) + C

4.7 Logarithmic Functions

∫ ln x dx = x ln x – x + C
∫ logₐx dx = (x ln x – x)/ln a + C

5. Integral Formulas for Special Functions

Apart from the common categories, some special functions also have direct integration formulas.

∫ sec x dx = ln|sec x + tan x| + C
∫ csc x dx = ln|csc x – cot x| + C
∫ tan x dx = ln|sec x| + C
∫ cot x dx = ln|sin x| + C

6. Practice Problem Using Integral Formulas

Question: Calculate ∫ 5x⁴ dx

Solution:

Use the power rule: ∫ xⁿ dx = (xⁿ⁺¹)/(n+1) + C
∫ 5x⁴ dx = 5 × (x⁵ / 5) + C
= x⁵ + C

Absolutely!
Here’s a complete study pack for your Integral Formulas topic that includes:

📘 Worksheet: Integral Formulas Practice

Basic Integration Formulas (Direct Application)
Evaluate the following:

$∫1 dx\int 1 \, dx$
$∫7 dx\int 7 \, dx$
$∫x4 dx\int x^4 \, dx$
$∫sin⁡x dx\int \sin x \, dx$
$∫cos⁡x dx\int \cos x \, dx$
$∫ex dx\int e^x \, dx$
$∫ax dx\int a^x \, dx$
$∫sec⁡2x dx\int \sec^2x \, dx$
$∫csc⁡2x dx\int \csc^2x \, dx$
$∫1x dx\int \frac{1}{x} \, dx$

Mixed Functions
Use substitution, splitting, or rearrangement if needed.

$∫(x3+5×2) dx\int (x^3 + 5x^2) \, dx$
$∫(3sin⁡x+4cos⁡x) dx\int (3\sin x + 4\cos x) \, dx$
$∫(ex+x2) dx\int (e^x + x^2) \, dx$
$∫(1x+x)dx\int \left(\frac{1}{x} + x\right) dx$
$∫sec⁡xtan⁡x dx\int \sec x \tan x \, dx$

Part C — Application Problems
Solve and write the final answer with $+C+C$ .

Find $F(x)F(x)$ if $dFdx=4×3+2x\frac{dF}{dx} = 4x^3 + 2x$ .
Find the function $yy$ if $dydx=cos⁡x−2sin⁡x\frac{dy}{dx} = \cos x – 2\sin x$ .
Find $∫5×4 dx\int 5x^4 \, dx$ .
Evaluate $∫3x dx\int \frac{3}{x} \, dx$ .
Find $∫(2ex+3)dx\int (2e^x + 3) dx$ .

📝 Practice Quiz: Integral Formulas Mastery

Instructions: Choose the correct answer. Each question carries 1 mark.

Q1. $∫xn dx\int x^n \, dx$ equals:
A) $xn+1n+1+C\frac{x^{n+1}}{n+1} + C$ (n ≠ -1)
B) $nxn−1+Cnx^{n-1} + C$
C) $xn+Cx^n + C$
D) $xn+1+Cx^{n+1} + C$

Q2. The integral of $sin⁡x\sin x$ is:
A) $cos⁡x+C\cos x + C$
B) $−cos⁡x+C-\cos x + C$
C) $−sin⁡x+C-\sin x + C$
D) $sec⁡x+C\sec x + C$

Q3. $∫sec⁡2x dx\int \sec^2x \, dx$ equals:
A) $cot⁡x+C\cot x + C$
B) $sec⁡x+C\sec x + C$
C) $tan⁡x+C\tan x + C$
D) $sin⁡x+C\sin x + C$

Q4. Which of the following is correct?
A) $∫1xdx=ln⁡∣x∣+C\int \frac{1}{x} dx = \ln|x| + C$
B) $∫exdx=ex+C\int e^x dx = e^x + C$
C) Both A and B
D) None of the above

Q5. $∫axdx\int a^x dx$ is equal to:
A) $axln⁡a+C\frac{a^x}{\ln a} + C$
B) $ax+Ca^x + C$
C) $ln⁡a+C\ln a + C$
D) $1ax+C\frac{1}{a^x}+C$

Q6. If $dydx=cos⁡x\frac{dy}{dx} = \cos x$ , then $y=y =$
A) $cos⁡x+C\cos x + C$
B) $sin⁡x+C\sin x + C$
C) $−cos⁡x+C-\cos x + C$
D) $−sin⁡x+C-\sin x + C$

Q7. The integral of $sec⁡xtan⁡x\sec x \tan x$ is:
A) $tan⁡x+C\tan x + C$
B) $csc⁡x+C\csc x + C$
C) $sec⁡x+C\sec x + C$
D) $−sec⁡x+C-\sec x + C$

Q8. Which formula represents integration as reverse differentiation?
A) $∫f′(x)dx=f(x)+C\int f'(x) dx = f(x) + C$
B) $ddxf(x)=∫f(x)dx\frac{d}{dx}f(x)=\int f(x) dx$
C) $∫f(x)dx=ddxf(x)\int f(x) dx = \frac{d}{dx}f(x)$
D) $f(x)=f'(x)+Cf(x) = f'(x) + C$

Q9. $∫csc⁡2xdx\int \csc^2x dx$ equals:
A) $−cot⁡x+C-\cot x + C$
B) $cot⁡x+C\cot x + C$
C) $−csc⁡x+C-\csc x + C$
D) $tan⁡x+C\tan x + C$

Q10. If $∫(2x+3)dx\int (2x+3) dx$ is calculated, the answer will be:
A) $2\times2+3x+C2x^2+3x+C$
B) $x2+3×22+Cx^2+\frac{3x^2}{2}+C$
C) $x2+3x+Cx^2+3x+C$
D) $x2+3+Cx^2+3+C$

✅ Key Takeaways

Integration is the reverse process of differentiation.
Mastering basic and advanced integral formulas is crucial for solving complex calculus problems.
Categorizing formulas by function type can help improve learning and recall.
Practicing problems regularly ensures better understanding and application.

Resources:

Vernier Caliper Least Count

A Vernier caliper is a precise measuring instrument used to measure small dimensions with high accuracy. The least count of a Vernier caliper refers to the smallest measurement it can accurately read. It is calculated as the difference between one main scale division and one Vernier scale division. Typically, the least count of a Vernier caliper is 0.02 mm or 0.001 inches. This value represents the tool’s precision and helps in taking more accurate measurements than standard rulers. Understanding the least count is essential for users who need precise measurements in scientific, engineering, or mechanical applications.

📑 Table of Contents

1. List of Basic Integral Formulas

2. Integral Formulas PDF

3. Classification of Integral Formulas