Ohm’s Law is a fundamental principle in electrical engineering that relates the three essential quantities in an electrical circuit: voltage, current, and resistance. It states that the current flowing through a conductor is directly proportional to the voltage applied across it and inversely proportional to the resistance of the conductor.
Understanding is crucial for comprehending the behavior of electrical circuits and designing safe and efficient systems. It forms the basis for many electrical calculations and serves as a foundation for more advanced concepts in electrical engineering.
Relationship Between Voltage, Current, and Resistance
Ohms Law establishes a clear relationship between voltage, current, and resistance in an electrical circuit. According to this law, the current (I) flowing through a conductor is equal to the voltage (V) across the conductor divided by its resistance (R), as expressed by the equation:
I = V / R
This equation implies that increasing the voltage will result in a larger current flow while increasing the resistance will decrease the current flow. It demonstrates the proportional and inverse relationship between these three quantities.
Understanding the relationship between voltage, current, and resistance is essential for analyzing and designing electrical circuits, as it allows engineers to predict the behavior of the system and make informed decisions regarding component selection and circuit optimization.
Water Pipe Analogy
A helpful analogy to understand Ohm’s Law is to compare it to the flow of water in a pipe.
Just as increasing the water pressure results in a higher flow rate, increasing the voltage in an electrical circuit leads to a greater current flow. Similarly, a narrower or clogged pipe restricts water flow, just as higher resistance in a circuit limits the current.
This analogy helps visualize the concepts of voltage, current, and resistance and how they interact in a circuit. It provides a relatable and intuitive way to understand Ohm’s Law and its applications in electrical systems.
Experimental Verification
- Experimental verification of Ohm’s Law involves setting up a simple circuit and measuring the voltage, current, and resistance to confirm the relationship predicted by the law.
- To verify Ohm’s Law, one can construct a circuit with a known resistor and a variable power supply. By measuring the voltage across the resistor using a voltmeter and the current flowing through the resistor using an ammeter, one can calculate the resistance using this Law and compare it to the known value.
Ohm’s Law Magic Triangle
The Ohm’s Law Magic Triangle is a graphical representation that helps simplify calculations involving voltage, current, and resistance. It provides a visual aid for quickly determining the missing value in equation I = V / R.
The triangle has three sides representing voltage (V), current (I), and resistance (R).
For example, if you need to find the current (I) and you know the voltage (V) and resistance (R), cover the “I” on the triangle, and you will see that the equation becomes I = V / R.
Ohm’s Law Solved Problems
- Problem: Calculate the current flowing through a resistor with a voltage of 12 volts and a resistance of 4 ohms.
- Solution: Using Ohm’s Law (I = V / R), the current (I) can be calculated as I = 12 V / 4 Ω = 3 A.
- Problem: Determine the resistance required to limit the current to 0.5 amps when a voltage of 9 volts is applied.
- Solution: Rearranging Ohm’s Law to solve for resistance (R = V / I), the resistance (R) is calculated as R = 9 V / 0.5 A = 18 Ω.
Calculating Electrical Power
Ohm’s Law not only relates voltage, current, and resistance but also provides a way to calculate electrical power in a circuit. The power (P) in watts can be determined using the formula:
P = V × I
Where V is the voltage across the circuit or component, and I is the current flowing through it.
By multiplying the voltage and current, engineers can determine the power dissipated or consumed in a circuit. This calculation is essential for selecting appropriate components, designing power systems, and ensuring circuit safety.
Ohm’s Law Pie Chart
- An Ohm’s Law pie chart is a graphical representation that illustrates the relationship between voltage, current, and resistance in a circuit. It visually demonstrates the proportionality and inverse relationship between these quantities.
- The size of each sector corresponds to the magnitude of the respective quantity. For example, if the voltage is larger than the current, the voltage sector will be larger than the current sector.
Matrix Table
- A matrix table is a tabular representation that organizes different combinations of voltage, current, and resistance values according to this Law. It serves as a handy reference for performing calculations and understanding the relationships between these quantities.
- The matrix table has columns for voltage (V), current (I), and resistance (R), and each row represents a specific combination of two known quantities. By locating the known values in the table, users can easily find the missing value using the corresponding formula.
- The Ohm’s Law matrix table simplifies calculations and eliminates the need for complex rearrangements of equations. It is a useful tool for both beginners and experienced engineers when working with this Law.
Applications
- Circuit Analysis: It is essential for analyzing electrical circuits, determining voltage drops, current distributions, and power dissipation, and verifying circuit behavior.
- Component Selection: Engineers use this Law to select resistors, capacitors, and other components based on desired voltage and current requirements.
- Circuit Design: It helps engineers design circuits that operate within safe limits, ensuring components do not exceed their rated voltage or current values.
- Power Calculations: By applying this Law, engineers can calculate power dissipation in resistors, and power consumption of electrical devices, and determine appropriate power ratings for components.
- Troubleshooting: It aids in diagnosing and troubleshooting electrical problems by analyzing voltage, current, and resistance measurements in circuits.
- Electrical Safety: Understanding this Law is crucial for ensuring electrical safety, preventing circuit overloads, and avoiding hazards caused by excessive current or voltage.
Limitations of Ohm’s Law
- Linearity: It assumes that the relationship between voltage, current, and resistance is linear. In reality, some components, such as diodes or transistors, may not follow this Law due to their nonlinear behavior.
- Temperature Dependency: It assumes constant resistance, but in some materials, resistance can vary with temperature. This can affect the accuracy of calculations in circuits with temperature-dependent resistors.
- Frequency Dependency: It applies to direct current (DC) circuits but may not fully account for the behavior of alternating current (AC) circuits. In AC circuits, additional considerations, such as impedance and reactance, come into play.
- Material Constraints: It assumes that the conducting material remains in its linear region of operation and does not change properties under varying voltages or currents. Non-ideal behavior of materials can introduce deviations from this Law.
Engineers must be aware of these limitations and consider them when working with non-ideal components or complex circuits.
Ohm’s Law Faqs:
Ohm's Law states that the current passing through a conductor is directly proportional to the voltage across it and inversely proportional to its resistance. Mathematically, it can be expressed as I = V / R. where I represents the current flowing through the conductor in Amperes (A), V denotes the voltage across the conductor in Volts (V), and R signifies the resistance of the conductor in Ohms (Ω).
It is based on the concept that electric current is the flow of charged particles (usually electrons) through a conductor. Conversely, if the resistance increases while keeping the voltage constant, the current decreases.
Ohm's Law is of paramount importance in electrical engineering and plays a crucial role in circuit analysis and design. Ohm's Law forms the foundation for many other electrical concepts and laws.
It finds practical applications in various areas, including electronics, power systems, telecommunications, and automotive engineering.
It involves three primary units: Amperes (A) for current, Volts (V) for voltage, and Ohms (Ω) for resistance. It is essential to use consistent units when applying this Law to ensure accurate calculations and analysis.
It apply to most passive electrical circuits, where the relationship between voltage, current, and resistance remains linear. However, it may not hold for complex circuits involving non-linear elements such as diodes and transistors.
While Ohm's Law is a fundamental principle, it has certain limitations. It assumes that the conductor's temperature remains constant and that the material's properties do not change significantly. In reality, factors like temperature variations and nonlinear behaviors can affect the accuracy of Ohm's Law in some cases.
Ohm's Law can be used to calculate power in a circuit. By substituting this Law equation (I = V / R) into the power formula, we can derive an alternative expression for power: P = (V^2) / R. This equation shows that power is directly proportional to the square of the voltage and inversely proportional to the resistance.
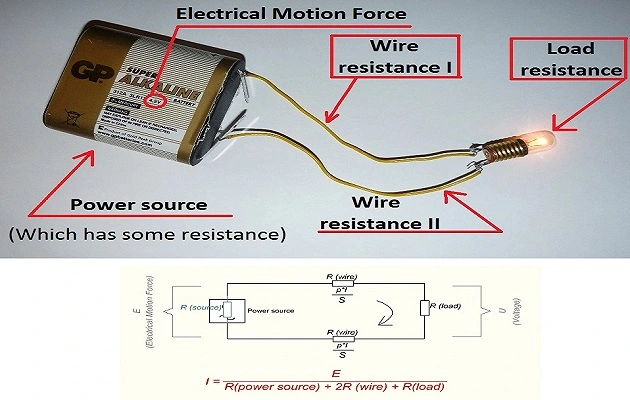
While this Law provides valuable insights into electrical circuits, it is crucial to consider certain practical limitations. For instance, it assumes ideal conditions and may not account for factors like wire resistance and voltage drops across connectors. In real-world applications, these factors can impact the accuracy of calculations based solely on Ohm's Law.
To apply this Law to a simple circuit problem, start by identifying the known values, such as the voltage or current. Use Ohm's Law equation (I = V / R) to calculate the unknown value, ensuring that the units are consistent throughout the calculation. By rearranging the equation, you can solve for the desired quantity.
Understanding this Law is crucial for ensuring circuit safety. This knowledge helps prevent electrical hazards and ensures the longevity of electrical systems.
It holds for most conductive materials under normal conditions. However, there are exceptions, such as materials exhibiting non-linear behavior or components like diodes that have specific voltage-current characteristics. In such cases, this Law alone may not accurately predict the behavior of the circuit.
Temperature can significantly impact the behavior of materials and, consequently, their resistance. As temperature changes, the resistance of a material can increase or decrease, affecting the application of this Law.
In series circuits, where components are connected sequentially, Ohm's Law can be used to calculate the total resistance and determine the current flowing through each component.
To verify this Law experimentally, you can set up a simple circuit with a known resistor and measure the voltage across it while varying the current.
It have several practical applications in everyday life. Additionally, it forms the basis for various electronic devices and systems we use daily.
To calculate resistor values using this Law, start by determining the desired current or voltage across the resistor. This process helps in selecting the appropriate resistor to achieve the desired circuit behavior.
When dealing with mixed units in this Law problems, it is crucial to ensure consistency throughout the calculation. Convert all values to a common unit before performing the calculations.
According to this Law, the resistance of a wire is directly proportional to its length and inversely proportional to its cross-sectional area (thickness). Longer wires have higher resistance, while thicker wires have lower resistance for the same material.
It applies to most conductive materials commonly used in electrical circuits, such as metals.
It is a fundamental component of circuit analysis techniques like Kirchhoff's Law. It provides the foundational relationship needed to apply Kirchhoff's laws effectively. What is Ohm's Law?
How does Ohm's Law work?
What is the significance of Ohm's Law?
How is Ohm's Law used in practice?
What are the units used in Ohm's Law?
Can Ohm's Law be applied to all types of circuits?
Are there any limitations to Ohm's Law?
How is Ohm's Law related to power?
Are there any practical limitations to using Ohm's Law?
How can I apply Ohm's Law to solve a simple circuit problem?
What is the role of Ohm's Law in circuit safety?
Are there any exceptions to Ohm's Law?
How does temperature affect the application of Ohm's Law?
How does Ohm's Law relate to series and parallel circuits?
How can I verify Ohm's Law experimentally?
What are some practical applications of Ohm's Law in everyday life?
How can I use Ohm's Law to calculate resistor values?
How can I solve Ohm's Law problems involving mixed units?
How do the length and thickness of a wire affect its resistance according to Ohm's Law?
Does Ohm's Law apply to all types of conductors?
How does Ohm's Law relate to circuit analysis techniques like Kirchhoff's laws?
To know more about Education Information.